In Post 86 I showed how the Greenhouse Effect can be viewed as resulting from a combined process of absorption of infra-red radiation by molecules of carbon dioxide (CO2), followed by re-emission of the same wavelength of radiation, but in a different random direction. The law that leads to this result is the principle of detailed balance. I also showed how this process resembled a scattering process, but with a much greater strength due to the significantly greater absorption cross-section (σa) of CO2 compared to its cross-section for Rayleigh scattering, σs. Therefore it could be modelled as a scattering process, but one with an appropriately large cross-section.
Then in Post 88 I showed how this combined process of absorption and re-emission resulted in absorption that decreased linearly with distance x into a gas of uniform density, rather than exponentially as predicted by the Beer-Lambert law. The importance of this result is that it demonstrates that the transmission of radiation through an absorbing gas will be greater than that predicted by the Beer-Lambert law, and so changes to the concentration of the absorbing gas will have a larger impact on the overall transmission than the Beer-Lambert law would predict.
Sadly this result has been questioned by some who have claimed that the scattering (or combined absorption and re-emission) approach is not valid as it does not follow from, or agree with, Schwarzschild's equation. This equation is possibly named after the German physicist Karl Schwarzschild (shown below), although it could actually be named after his son Martin Schwarzschild who worked in the field of stellar evolution. In this post I will show that this claim of non-validity is not true and that Schwarzschild's equation leads to the exact same results as I outlined in Post 88.
Fig. 91.1: Karl Schwarzschild (1873-1916)
The Model
In order to understand the Greenhouse Effect we need to consider the radiation flows through a thin layer of gas of thickness δx at some altitude x, and then extend this to model to the gas as a whole. In particular, we need to consider radiation flows through the gas from opposing directions. This is because each thin layer of the gas only absorbs a small fraction of radiation passing through it. Most of the rest is then absorbed by subsequent layers above the initial layer. However, after absorption the radiation is re-emitted and the re-emission process occurs equally in both directions (up and down). This means that some of this emitted radiation will travel in a downwards direction and reheat the first layer from the opposite direction. This is why the Beer-Lambert law fails.
In order to model this process we need to consider radiation fluxes in both directions as shown in Fig. 91.2 below. Let's assume that the gas has a concentration or particle density n(x), and each molecule of it has an absorption cross-section σa. If radiation of intensity Iu(x) travels in an upward direction from a hot surface and enters the gas layer from below, as shown in Fig. 91.2 below, a proportion of it will be absorbed by the gas. This in turn will heat the gas and cause it to emit radiation in both an upwards and a downwards direction. The result will be that the intensity of upward radiation leaving the gas layer will change by an amount δIu(x).
Fig. 91.2: A schematic illustration of how scattering, absorption and thermal emission within each thin layer of the atmosphere alters the intensities of the upwelling (Iu) and downwelling (Id) radiation.
The upwelling radiation will then go on to interact with gas above the layer shown in Fig. 91.2 and thus heat this gas as well. This gas will then re-emit radiation, some of it in a downward direction, and this downwelling radiation Id(x) will then also pass through our original layer of gas. It in turn will be partially absorbed, heating the gas and causing it to radiate. The net result is that the intensity of the downwelling radiation leaving the gas layer will also change, in this case by an amount δId(x). So far this model is identical to the one outlined in Post 88. The next step is to consider the changes of intensity, δIu(x) and δId(x), using Schwarzschild's equation and the Planck function, B(λ,T), where λ is the wavelength of the radiation and T is the temperature of the gas in kelvins at this height x. In the following discussion I will consider the absorption and re-emission of radiation at a fixed wavelength λ.
The Proof
As the upwelling radiation Iu(x) at a fixed wavelength λ passes through the thin layer of gas of thickness δx a small amount of it will be absorbed by the gas. This amount will be proportional to the number of molecules encountered per unit area, n(x)δx, their absorption cross-section, σa, and the amount of radiation in the original flux, Iu(x). The result is that the transmitted intensity will decrease by an amount equal to Iu(x)σan(x)δx.
At the same time the thin layer of gas will be emitting radiation in both the upward and downward direction due to its temperature T. This temperature will in turn vary with height x, so both B(λ,T) and Iu(x) will vary with x. The intensity emitted in each direction is given by the Planck function, B(λ,T), multiplied by the number of molecules per unit area in the layer, n(x)δx, and the emission cross-section, σe. The result of this emission process is that the transmitted intensity above the layer will increase by an amount equal to B(λ,T)σen(x)δx.
Combining these two effects of emission and absorption gives the total change in intensity of the upwelling radiation
δIu(x) = [B(λ,T)σe - Iu(x)σa]n(x)δx
(91.1)
This is Schwarzschild's equation. However, it is only half the solution because there is a similar equation that can be derived for the changes to the downwelling radiation Id(x) in the thin layer at the same wavelength λ. Thus the resulting equation will be
δId(x) = [B(λ,T)σe - Id(x)σa]n(x)δx
(91.2)
Finally, there is one other consideration we must take into account: energy conservation at wavelength λ. The total energy flowing into the layer of gas must match the energy flowing out. This means that
δIu(x) + δId(x) = 0
(91.3)
and so
[ Iu(x) + Id(x) ]σa = 2B(λ,T)σe
(91.4)
We can now eliminate B(λ,T)σe from Eq. 91.1 and Eq. 91.2 and turn both equations into first order differential equtions. However, because the change in downwelling radiation Id(x) in Fig. 91.2 is defined to be positive for changes of x in a negative direction the δx term must be negative, and therefore the differential term dId/dx = - δId/δx.This means that
It then turns out that Eq. 91.5 is identical to Eq. 88.5 in Post 88. So if the equations are the same and the boundary conditions are the same, then the solutions must be the same as in Post 88. So even using Schwarzschild's equation as our starting point we end up with the same result.
Finally, we can write Schwarzschild's equation as two differential equations, one for Iu(x) and one for Id(x). From Eq. 91.1 we get the obvious result
(91.6)
The equivalent equation for Id(x) is similar, but requires some explanation.
In Eq. 91.7 the order of the I(x)σa and B(λ,T)σe terms to the right of the equality sign have been reversed compared to Eq. 91.6. This is because the downwelling radiation, Id(x), is flowing in the negative direction through the thin gas layer. So for Iu(x) the radiation term B(λ,T)σe is directed in the same direction as Iu(x) so it must be positive, while the absorption in the gas layer must leads to a lower value of Iu(x) above the layer than below. In the case of Id(x) the reverse it true in both cases, hence the reversal of sign for both terms.
Finally, it should be noted that conditions of thermal equilibrium generally require the cross-sections σa and σe to be equal. This is particularly true for black body radiation where a black body at constant temperature must emit what it absorbs, otherwise it will gain or lose energy, and thus not be at constant temperature.
Implications
If we now apply the above results to a real system and consider the case of a column of gas of thickness L, and uniform density n that is independent of x, the model outlined above means that the upwelling radiation will vary with x through the gas as (see Post 88)
(91.8)
where Io is the intensity of radiation entering the gas at x = 0, while the downwelling radiation will vary as
(91.9)
The difference between Iu(x) and Id(x) will be constant (ΛIo), as required by energy conservation, and will be equal to the amount transmitted at the end of the column (i.e. at x = L), so
(91.10)
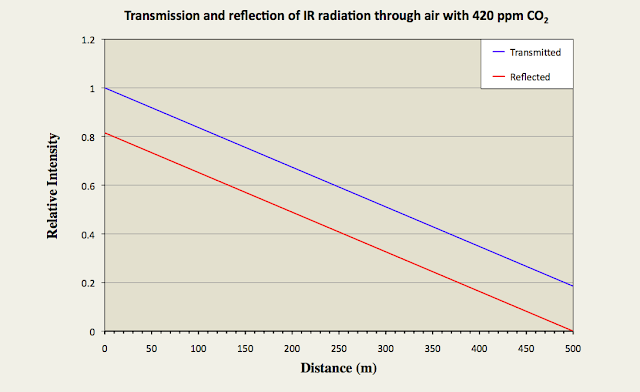
In Fig. 91.3 below I have suggested what Iu(x) and Id(x) might look like through a 500 metre long horizontal column of air with 420 ppm of CO2 when it is irradiated with 15 µm infra-red radiation from one end. It should be noted, though, that this theoretical data is based on an estimated value for the scattering cross-section of the CO2 molecules that could be almost a factor of ten too small. Nevertheless, it still illustrates qualitatively how the transmission of 15µm infra-red radiation changes through the gas, and also how the Greenhouse Effect (as defined by the relative intensity of the reflected radiation at x = 0) is related to this transmission (see the red curve in Fig. 91.3).
Fig. 91.3: The relative intensities of transmitted (Iu) and reflected (Id) radiation along a 500 metre column of air containing 420 ppm of CO2 with an absorption cross-section of 1.6 x 10-24 m2.
The graph in Fig. 91.3 suggests that a 500 metre column of air will transmit less than 18% of the incident radiation and reflect back the rest. The question is: is this more or less than that predicted by the Beer-Lambert law? The answer to this can be seen in Fig. 91.4 below.
Fig. 91.4: The relative intensities of radiation transmitted through a column of air of length L containing 420 ppm of CO2 with an absorption cross-section of 1.6 x 10-24 m2 based on two different models: (i) a backscattering model described by Eq. 91.10 (blue curve), and (ii) the Beer-Lambert law (red curve).
The blue curve in Fig. 91.4 demonstrates how the transmission through a column of air would decrease with the length of the column, L, if half of the absorbed radiation is backscattered or re-emitted in the reverse direction and half is re-emitted in the forward direction. The result is a curve that decays with L in accordance with Eq. 91.10. The red curve on the other hand shows how the transmission would change if it followed the (commonly accepted) Beer-Lambert law where the transmitted intensity varies with L as
(91.11)
What is clear is that the Beer-Lambert law underestimates the transmission for large values of L, and in effect predicts that there is no significant transmission beyond 400 metres. The blue curve shows that this is not the case with over 10% transmission for a column of length 1000 metres. In practice this means that the Beer-Lambert law will massively underestimate the impact of future increases in CO2 levels because it will assume that the backscattering is already operating at close to 100% or saturation with 420 ppm of CO2. Yet clearly it is not. In fact even at 10 km (the effective thickness of Earth's atmosphere) there is still more than 1% transmission. While this is small, it is not insignificant, particularly when considering the relatively small changes to the overall size of the Greenhouse Effect that are needed to create a warming of 1.5°C.
Caveats
The only condition I have applied to the proof and discussion described here is that set out in Eq. 91.4, which basically demands that each layer of the gas radiates the same amount of energy at each wavelength as it absorbs from the two fluxes, Iu(x) and Id(x). This will be true provided all the heat of wavelength λ entering the gas originates from the Earth's surface at the bottom of Fig. 91.2, or at the very top of the atmosphere. In which case, the exact functional forms of Iu(x) and Id(x) will depend only on the functional form of n(x) and the boundary conditions. However, at the top of the atmosphere this condition will not be satisfied as some of the incoming ultraviolet radiation from the Sun is absorbed there before it reaches the ground and so gets converted to infra-red radiation within the upper atmosphere. This means that Eq. 91.4 is no longer satisfied for each and every infra-red wavelength of interest within the gas.
In my next blog post I will consider a number of distinct and important examples to demonstrate how dependent the transmission is on the density profile of the gas, n(x), and also on the location of the heat source(s). These examples will also show that even when it sometimes looks like there is no Greenhouse Effect in operation, it is still there.





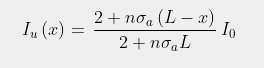



Hi Climate Detector,
ReplyDeleteGreetings,
You have touched a very important topic that is Beer-Lambert's law and reality in the atmosphere.
In Fig. 91.3, you said that the transmitted intensity is ~18%. Is not 18% of the incoming intensity being absorbed before it reflects off the ground?
Thanks